Volume of Composite Figures Worksheets⁚ A Comprehensive Guide
This guide explores the use of worksheets for calculating the volume of composite figures. PDF worksheets offer practice problems ranging in difficulty, from basic shapes to more complex 3D solids. These resources aid in mastering volume calculations and real-world applications.
Introduction to Composite Figures
Composite figures, in the context of geometry, refer to three-dimensional shapes formed by combining two or more basic geometric solids. These solids can include cubes, rectangular prisms, cylinders, cones, spheres, pyramids, and more. Understanding composite figures is crucial for developing spatial reasoning skills and applying volume calculation principles to real-world scenarios. Worksheets focusing on composite figures often present students with diagrams of these complex shapes, requiring them to deconstruct the figure into its simpler components to determine the overall volume. This process involves identifying the individual shapes, calculating their respective volumes using appropriate formulas, and then adding or subtracting those volumes depending on the arrangement of the shapes within the composite figure. The ability to break down complex shapes into simpler ones is a key skill in problem-solving and spatial reasoning.
Types of Composite Figures Found in Worksheets
Worksheets on composite figure volumes typically feature a variety of combinations of basic 3D shapes. Common examples include figures combining rectangular prisms (boxes) with cubes, or prisms combined with cylinders. More challenging worksheets might include pyramids and cones in conjunction with prisms or cylinders, creating irregular shapes requiring a deeper understanding of volume calculation techniques. Some worksheets focus solely on prisms and cubes, building progressively more complex arrangements to reinforce foundational skills. Others introduce spheres and hemispheres to enhance problem-solving complexity. The variety ensures students encounter diverse challenges, promoting a comprehensive understanding of volume calculations across different geometric forms. The level of complexity increases gradually across different grade levels, starting with simpler combinations and progressing to more intricate, multi-shape figures. This gradual progression is key to building a strong foundation in spatial reasoning and volume calculation.
Calculating Volume⁚ Basic Principles
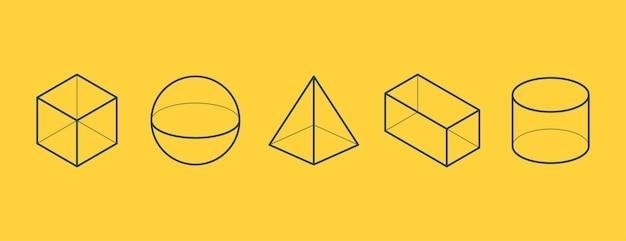
Calculating the volume of composite figures hinges on understanding the volume formulas for individual 3D shapes. For rectangular prisms, the formula is length × width × height. Cubes, a special case of rectangular prisms, use the same formula with all sides equal. Cylinders utilize the formula πr²h, where ‘r’ is the radius and ‘h’ is the height. For pyramids, the formula is (1/3) × base area × height, requiring the calculation of the base area first. Cones employ a similar formula⁚ (1/3)πr²h. Spheres use (4/3)πr³. Understanding these individual formulas is crucial before tackling composite figures. Worksheets often provide a reference sheet of these formulas, or the students are expected to have them memorized. The key to solving problems involving composite figures lies in recognizing the individual shapes that make up the composite figure and applying the appropriate formula to each component shape.
Additive Volume⁚ Combining Simple Shapes
Many composite figures are formed by combining simpler shapes like rectangular prisms, cubes, cylinders, cones, pyramids, and spheres. Additive volume problems involve calculating the volume of each individual shape and then summing these individual volumes to find the total volume of the composite figure. Worksheets often present diagrams clearly showing the distinct shapes within the composite figure, making identification easier. For example, a figure might consist of a rectangular prism sitting atop a cube. The student would calculate the volume of the prism and the volume of the cube separately, using the appropriate formulas, and then add the two volumes together for the final answer. This approach simplifies complex problems by breaking them down into smaller, manageable calculations. Success hinges on accurately identifying each component shape and correctly applying the corresponding volume formula. Practice problems increase in complexity, sometimes involving three or more combined shapes. Clear labeling of dimensions on the diagrams is essential for accurate calculations.
Subtractive Volume⁚ Finding the Volume of Irregular Shapes
Determining the volume of irregular composite shapes often involves a subtractive approach. These shapes can be visualized as a larger, simpler shape with a smaller shape removed. Worksheets frequently present these scenarios with diagrams showing a larger shape (e.g., a rectangular prism) with a smaller shape (e.g., a cylinder) cut out from its interior. To find the volume of the irregular shape, students first calculate the volume of the larger, encompassing shape. Next, they calculate the volume of the smaller, removed shape. Finally, they subtract the volume of the smaller shape from the volume of the larger shape; This difference represents the volume of the irregular composite figure. This method requires careful attention to detail, ensuring accurate measurement of all relevant dimensions for both shapes. The process helps students understand how to apply volume formulas strategically and conceptually. The worksheets often progress from simple subtractive problems to more complex scenarios involving multiple subtractions or combinations of additive and subtractive methods. Mastering this technique is crucial for tackling real-world problems involving irregularly shaped objects.
Worksheets for Different Grade Levels
The complexity of volume of composite figures worksheets varies significantly across grade levels. Elementary school worksheets (grades 3-5) typically focus on simpler composite shapes composed of two or three rectangular prisms. These problems often involve straightforward addition of volumes, with clear diagrams and step-by-step instructions guiding students. As students progress to middle school (grades 6-8), worksheets introduce more complex shapes, including triangular prisms, cylinders, and cones. Problems might involve both addition and subtraction of volumes, requiring a deeper understanding of geometric formulas. High school worksheets (grades 9-12) present even more challenging scenarios. These often include irregular shapes requiring multiple subtractions or the combination of additive and subtractive methods. Students at this level are expected to demonstrate a strong grasp of various 3D shapes and their respective volume formulas. Moreover, high school worksheets frequently incorporate more complex calculations, potentially involving decimals, fractions, and algebraic expressions within the problem-solving process. The availability of online resources and printable PDFs caters to diverse learning styles and educational settings, ensuring that students at all levels can access appropriate materials.
Practice Problems⁚ Increasing Difficulty
Effective worksheets on calculating the volume of composite figures employ a carefully structured progression of problem difficulty. Initial problems typically involve simple composite shapes, such as two rectangular prisms joined together, allowing students to focus on the fundamental concept of adding individual volumes. Subsequent problems gradually increase in complexity, introducing more irregular shapes and requiring students to apply a broader range of geometric formulas. This might involve incorporating triangular prisms, cylinders, or cones into the composite figure, demanding a deeper understanding of different volume calculation methods. Worksheets often include problems that necessitate the subtraction of volumes, such as finding the volume of a shape with a smaller shape removed from its interior. This step introduces the concept of subtractive volume, a crucial skill for handling more complex composite figures. The most advanced problems may combine additive and subtractive methods, requiring students to break down a complex shape into several simpler components, calculate their individual volumes, and then combine or subtract them accordingly. This layered approach ensures that students build a strong foundation before tackling increasingly challenging problems, fostering a comprehensive understanding of composite volume calculations.
Real-World Applications of Composite Volume
Understanding composite volume extends far beyond the classroom, finding practical applications in various real-world scenarios. Consider the construction of a building⁚ architects and engineers utilize composite volume calculations to determine the amount of material needed for complex structures composed of different shapes. Similarly, in manufacturing, calculating the volume of irregularly shaped components is vital for optimizing material usage and production processes. The design of storage containers, such as shipping crates or specialized packaging, frequently involves composite shapes, and accurate volume calculations ensure efficient use of space and prevent wasted resources. In the field of landscaping, determining the volume of soil or gravel required for a project often necessitates calculating the volume of composite figures. Even in everyday life, tasks like estimating the amount of water a uniquely shaped swimming pool can hold or calculating the volume of an oddly shaped storage bin in a garage rely on the principles of composite volume. These real-world examples emphasize the practical relevance of mastering composite volume calculations, transforming an abstract mathematical concept into a valuable and applicable skill.
Using Formulas for Different 3D Shapes
Successfully tackling volume of composite figures worksheets hinges on a solid grasp of the volume formulas for individual 3D shapes. These worksheets often incorporate a variety of shapes, necessitating familiarity with formulas for common solids. For rectangular prisms, the formula is length x width x height. Cylinders require the application of πr²h, where ‘r’ represents the radius and ‘h’ the height. Calculating the volume of spheres utilizes (4/3)πr³. Pyramids demand a slightly more complex formula⁚ (1/3)Bh, with ‘B’ representing the area of the base and ‘h’ the height. Cones, similarly, require (1/3)πr²h. Worksheets may present combinations of these shapes, requiring a stepwise approach. First, identify each individual shape within the composite figure. Then, apply the appropriate formula to each component, calculating individual volumes. Finally, add or subtract these individual volumes as dictated by the geometry of the composite figure—additive for shapes combined and subtractive for shapes removed from a larger solid. This systematic approach, coupled with accurate formula application, is key to mastering these worksheets.
Step-by-Step Solutions and Examples
Effective volume of composite figures worksheets frequently incorporate detailed, step-by-step solutions and examples. These serve as invaluable learning tools, guiding students through the problem-solving process. A well-structured worksheet will typically present a problem involving a composite figure, clearly outlining the individual shapes within the larger structure. The solution will then break down the calculation into manageable steps. This might involve first identifying each individual shape (e.g., rectangular prism, cylinder), then calculating the volume of each individual component using the appropriate formula. The next step would demonstrate how to combine (additive volume) or subtract (subtractive volume) the individual volumes to arrive at the final answer for the entire composite figure. Illustrative diagrams often accompany these step-by-step solutions, visually representing each stage of the calculation. These visual aids are particularly helpful in clarifying the process of breaking down complex composite figures into simpler components. The inclusion of multiple worked examples allows students to practice applying the techniques to different types of composite figures and varying levels of complexity, building confidence and competence.
Common Mistakes and How to Avoid Them
Many students encounter common pitfalls when calculating the volume of composite figures. One frequent error involves incorrectly identifying the individual shapes within the composite figure. Carefully examining the diagram and understanding the spatial relationships between the components is crucial. Another mistake is misapplying the appropriate volume formulas. Students should ensure they are using the correct formula for each individual shape (e.g., rectangular prism, cylinder, cone) before proceeding with calculations. A third common error arises from incorrect unit conversions. Pay close attention to the units used in the problem and ensure consistency throughout the calculation. Forgetting to add or subtract volumes correctly when dealing with additive or subtractive composite shapes is another prevalent issue. A clear understanding of the spatial relationship between the shapes is critical for accurate calculation. Finally, rounding errors can lead to inaccurate final answers. It’s important to maintain precision in calculations and only round the final answer to the appropriate number of significant figures. Worksheets addressing these common errors, through examples and explanations, are invaluable in helping students develop accurate and efficient problem-solving strategies. Careful attention to detail and a methodical approach will significantly reduce the occurrence of these mistakes.
Utilizing Online Resources and PDFs
The internet provides a wealth of resources for practicing volume calculations of composite figures. Numerous websites offer free printable worksheets in PDF format, catering to various grade levels and skill sets. These PDFs often include a range of problems, from simple exercises involving two combined shapes to more complex scenarios with multiple components. Many online resources provide answer keys, allowing students to self-check their work and identify areas needing improvement. Interactive online tools and tutorials can supplement these worksheets, offering dynamic visual aids and step-by-step guidance. These interactive resources can help students visualize the process of breaking down complex figures into simpler shapes, which is essential for accurate volume calculations. Furthermore, some websites offer customized worksheets, allowing educators to tailor the difficulty and content to meet the specific needs of their students. The availability of both printable and digital versions provides flexibility in how students can access and utilize these valuable resources. Remember to carefully review the source of any online resource to ensure its accuracy and reliability before using it for educational purposes. By combining the benefits of printable worksheets and interactive online tools, students can build a strong understanding of calculating the volume of composite figures.
Answer Keys and Self-Assessment
Many volume of composite figures worksheets available online, particularly those in PDF format, include answer keys. These keys are invaluable for students to check their work and identify any mistakes they may have made. The immediate feedback provided by an answer key allows for self-assessment, enabling students to pinpoint specific areas where they need further practice or clarification. This self-directed learning approach fosters independence and encourages students to take ownership of their learning process. By comparing their answers to the provided key, students can gain a deeper understanding of the concepts and techniques involved in calculating the volume of composite figures. The availability of answer keys also allows educators to save time on grading, freeing up more time to provide individualized support to students who are struggling. However, it’s crucial to emphasize the importance of understanding the underlying principles, rather than simply focusing on obtaining the correct answer; Students should aim to understand the steps involved in each problem, and use the answer key as a tool to identify and correct errors in their reasoning, not just to check for numerical accuracy. This process of self-assessment, guided by the answer key, ultimately enhances learning and reinforces the concepts related to composite figure volumes.